We have shared Quadratic Equations Class 10th maths notes to help the students better understand the chapter along with the ncert solutions of Quadratic equations class 10th to give them solutions and all the tips to solve a maximum number of questions at their fingertips.
Quadratic Equations Class 10 Maths: Introduction
Identification of quadratic equations
Example: Check whether the following are quadratic equations or not.
(i) (2x + 3)2 = 12x + 3
(ii) x (x + 3) = (x + 1) (x – 5)
Solution:
It is of the form ax2 + bx + c = 0, where a = 4, b = 0 and c = 6
Therefore, the given equation is a quadratic equation
It is not of the form ax2 + bx + c = 0, since the maximum power (or degree) of
the equation is 1.
Therefore, the given equation is not a quadratic equation.
Express has given situation mathematically
Example 1:
An express train takes 2 hours less than a passenger train to travel a distance of
240 km. If the average speed of the express train is 20 km/h more than that of
a passenger train, then form a quadratic equation to find the average speed of
the express train?
Solution:
Let the average speed of the express train be x km/h.
Since it is given that the speed of the express train is 20 km/h more than that
of a passenger train,
Therefore, the speed of the passenger train will be x −20 km/h.
Also, we know that
Time is taken by the express train to cover 240 km =
Time is taken by the passenger train to cover 240 km =
And the express train takes 2 hours less than the passenger train. Therefore,
This is the required quadratic equation.
Solution of Quadratic Equation by Factorization Method
If we can factorize, where a ≠ 0, into a product of two
linear factors, then the roots of this quadratic equation can be
calculated by equating each factor to zero.
Example:
Find the roots of the equation, , by factorization.
Solution:
or
Therefore, and are the roots of the given quadratic equation.
Solution of Quadratic Equation by completing the square
A quadratic equation can also be solved by the method of completing
the square.
Example:
Find the roots of the quadratic equation, , by the
method of completing the square.
Solution:
Therefore, –2 and are the roots of the given quadratic equation.
Quadratic Formula to find the solution of a quadratic equation:
The roots of the quadratic equation, , are given
by,
Example:
Find the roots of the equation, , if they exist, using the
quadratic formula.
Solution:
Here, a = 2, b = –3, c = –4
The roots of the given equation are given by.
The roots are –4 and.
Nature of roots of Quadratic Equation
For the quadratic equation, the
discriminant ‘D’ is defined as
The quadratic equation has
1. two distinct real roots, if
2. two equal real roots, if
3. has no real roots, if
Example: Determine the nature of the roots of the following
equations
(a)
(b)
Solution:
(a) Here, a = 2, b = 5, c = –117
Therefore, the roots of the given equation are real and
distinct.
(b) Here, a = 3, b = 5, c = 6
Therefore, the roots of the given equation are not real.
NCERT Solutions For Class 10 Maths Chapter 4 Quadratic Equations Ex 4.1
Ex 4.1 Class 10 Maths Question 1.
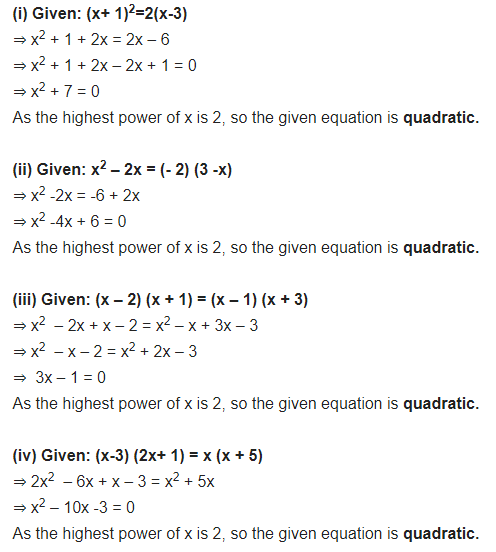
Check whether the following are quadratic equations:
(i) (x+ 1)2=2(x-3)
(ii) x – 2x = (- 2) (3-x)
(iii) (x – 2) (x + 1) = (x – 1) (x + 3)
(iv) (x – 3) (2x + 1) = x (x + 5)
(v) (2x – 1) (x – 3) = (x + 5) (x – 1)
(vi) x2 + 3x + 1 = (x – 2)2
(vii) (x + 2)3 = 2x(x2 – 1)
(viii) x3 -4x2 -x + 1 = (x-2)3
Solution:
Ex 4.1 Class 10 Maths Question 2.
Represent the following situations in the form of quadratic equations:
(i) The area of a rectangular plot is 528 m2. The length of the plot (in meters) is one more than twice its breadth. We need to find the length and breadth of the plot.
(ii) The product of two consecutive positive integers is 306. We need to find the integers.
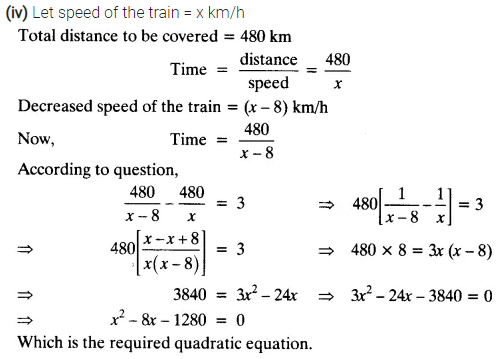
(iv) A train travels a distance of 480 km at a uniform speed. If the speed had been 8 km/h less, it would have taken 3 hours more to cover the same distance. We need to find the speed of the train.
Solution:

Conclusion
We have shared all about Quadratic Equations class 10th Maths to help out the students with the Ncert solutions for class 10th Quadratic equations as per the revised syllabus of the CBSE board.
Related Articles

